Probability Inflation: The Risk Of Ignoring Batting Average – Part 2

In my last post, I discussed how the probability of success in investing is grossly overestimated compared to historical batting average (funds assume 75% of success vs. their batting average of 55%). This causes miscalculations in portfolio allocation. In this post, I will discuss how Alpha Theory has been partially controlling for this phenomenon through our proprietary probability-weighted return calculation (Alpha Theory Probability-Weighted Return). Our method basically averages the arithmetic and geometric probability weighted returns. Note the difference between the Arithmetic and Geometric returns below.
‍

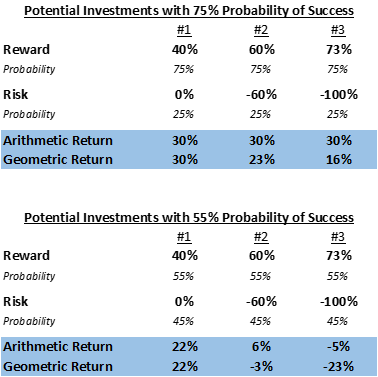
Geometric return more heavily weights the downside node and reduces the probability-weighted return for assets with high probabilities of extreme loss (see “Which Way is Up?” for further explanation). Not only does the geometric adjustment highlight the same gradation of the lower probabilities between the 75% and 55% arrays, it also is more intuitive to a portfolio manager. When surveyed, we found that portfolio managers would not size the top three positions equally when only shown the arithmetic return. There is an inherent heuristic that portfolio managers employ to control for this phenomenon. The problem is that heuristics are easily miscalculated and inconsistently applied.
‍

Alpha Theory uses a hybrid Arithmetic/Geometric return to calculate the Alpha Theory Probability-Weighted Return. This allows managers to properly account for portfolio impact and avoid heuristics. Downside is more impactful than upside in a compounding vehicle like a portfolio, so overemphasis of downside is practical. To properly allocate capital, it is critical for funds to first, make explicit forecasts of price targets and probability and then second, properly account for the asymmetry of upside and downside. This is difficult (impossible really) to accomplish without a systematic approach and we encourage every fund to capture and calculate risk-adjusted return for every investment in their portfolio.
‍

In portfolio management, preventing loss is paramount. Using realistic probabilities, more closely in line with the fund’s historical batting average, and Alpha Theory Probability-Weighted Return, will properly skew the portfolio towards those assets that have large asymmetry and little downside. In a compounding vehicle, like a portfolio, avoiding these “bad bets” will generate higher long-term geometric expected return, the ultimate goal of portfolio management.